Which Type Of Scale Would Most Likely Be Utilized To Draw A Map Of The United States?​

3. Calibration and Projections

Laura Matson and Melinda Kernik

Scale and projections are ii cardinal features of maps that unremarkably exercise not get the attending they deserve. Calibration refers to how map units relate to real-world units. Projections bargain with the methods and challenges around turning a three-dimensional (and sort of lumpy) earth into a two-dimensional map.

This chapter will introduce you to:

• Calibration and ways of telling the map user what the map is measuring on the ground
• Projection mechanics, types of projections, and their characteristics

By the end of this chapter, y'all should be able to read map scales and identify mutual projections along with their bones features and uses.

The world is vast. The earth's surface has an area of over 500 million km^two and any movie of the earth that you can easily carry can only testify general outlines of continents and countries. When we visually represent a region of the globe on a map, we must reduce its size to fit within the boundaries of the map. Map scale measures how much the features of the earth are reduced to fit on a map; or more precisely, map calibration shows the proportion of a given distance on a map to the corresponding distance on the ground in the real world.

Map calibration is represented by a representative fraction, graphic scale, or verbal description.

Map scales. A map can have a representative fraction, graphic scale, or verbal clarification that all mean the same thing. ^[1]

Representative fraction. The most commonly used measure of map scale is the representative fraction (RF), where map scale is shown as a ratio. With the numerator e'er set to 1, the denominator represents how much greater the distance is in the world. The figure beneath shows a topographic map with an RF of one:24,000, which means that one unit of measurement on the map represents 24,000 units on the ground. The representative fraction is accurate regardless of which units are used; the RF tin can exist measured equally 1 centimeter to 24,000 centimeters, one inch to 24,000 inches, or any other unit.

Representative fraction. Representative fraction and scale bars from a Us Geological Survey (USGS) topographic map. This topographic map has an RF of ane:24,000, which means that one unit of measurement on the map represents 24,000 units on the ground. ^[2]

Graphic scale. Scale bars are graphical representations of altitude on a map. The effigy has scale confined for ane mile, 7000 anxiety, and one kilometer. One of import advantage of graphic scales is that they remain true when maps are shrunk or magnified.

Verbal clarification. Some maps, especially older ones, employ a verbal clarification of scale. For case, it is common to see "ane inch represents 1 kilometer" or something like written on a map to give map users an thought of the scale of the map.

Map makers utilize the term scale to describe maps as beingness pocket-size-scale or large-scale. This description of map calibration equally large or pocket-size tin seem counter-intuitive at first. A 3-meter by 5-meter map of the United States has a small map scale while a UMN campus map of the same size is large-scale. Scale descriptions using the RF provide one fashion of considering calibration, since 1:1000 is larger than 1:1,000,000. Put differently, if nosotros were to change the scale of the map with an RF of 1:100,000 so that a section of road was reduced from i unit to, say, 0.ane units in length, nosotros would have created a smaller-calibration map whose representative fraction is i:1,000,000.

When we talk well-nigh large- and modest-scale maps and geographic data, and then, nosotros are talking about the relative sizes and levels of detail of the features represented in the data. In full general, the larger the map scale, the more than particular that is shown.

The extent of a map describes the area visible on the map, while resolution describes the smallest unit of measurement that is mapped. Y'all can think of the extent as describing the region to which the map is zoomed. The extent of the map below is national as information technology encompasses the contiguous U.s.a., while the resolution is the state, because states are the finest level of spatial detail that we can see.

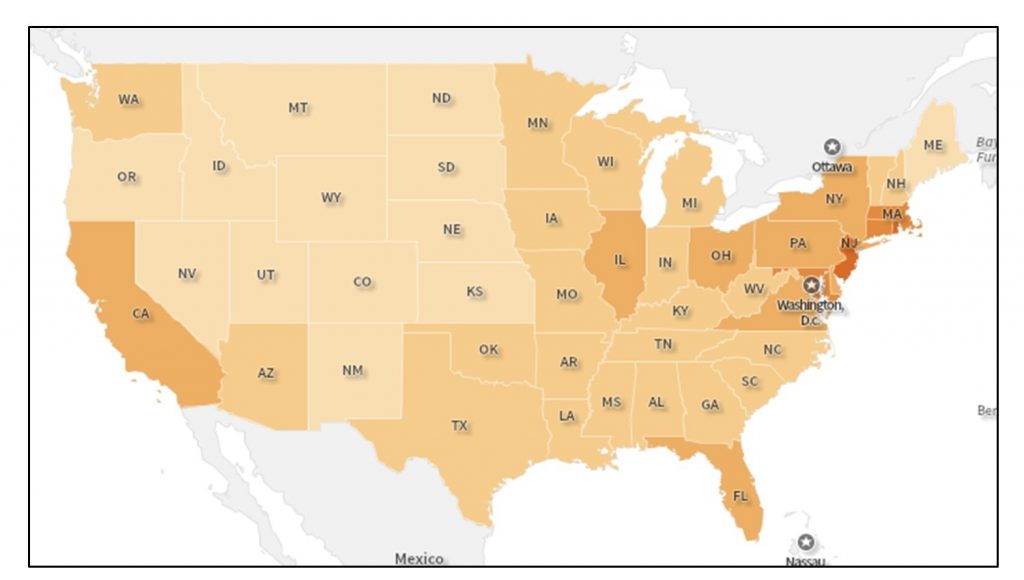
Map resolution and extent. This map shows a national extent and a state resolution. The extent of the map is national while the resolution is at the country level considering they are the finest level of spatial detail that we can see. ^[3]

We often cull mapping resolutions intentionally to make the map easier to understand. For example, if nosotros tried to display a map with a national extent at the resolution of demography blocks, the level of item would be so fine and the boundaries would exist then pocket-size that it would be hard to understand annihilation near the map. Balancing extent and resolution is often one of the most important and difficult decisions a cartographer must brand. The figure below offers two more examples of the difference between extent and resolution.

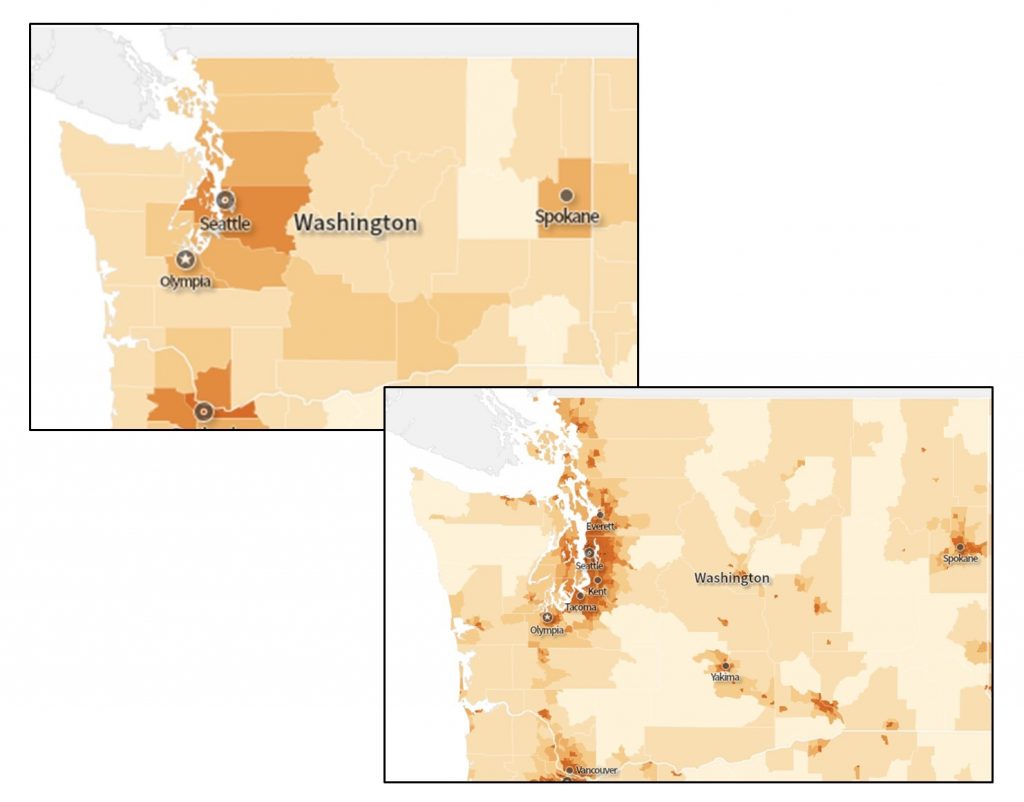
More than map extent and resolution. Maps showing an extent of the Pacific Northwest. The top prototype has a spatial resolution of the county and the bottom has a spatial resolution of demography tracts. ^[4]

Locations on the world's surface are measured in terms of coordinates, a gear up of ii or more than numbers that specifies a location in relation to some reference system. The simplest arrangement of this kind is a Cartesian coordinate system, named for the 17^th century mathematician and philosopher René Descartes. A Cartesian coordinate organisation, like the one beneath, is only a grid formed by putting together two measurement scales, ane horizontal (x) and ane vertical (y). The point at which both x and y equal zero is called the origin of the coordinate system. In the figure, the origin (0,0) is located at the center of the grid (the intersection of the ii assuming lines). All other positions are specified relative to the origin, as seen with the points at (three, ii) and (-4, -1)

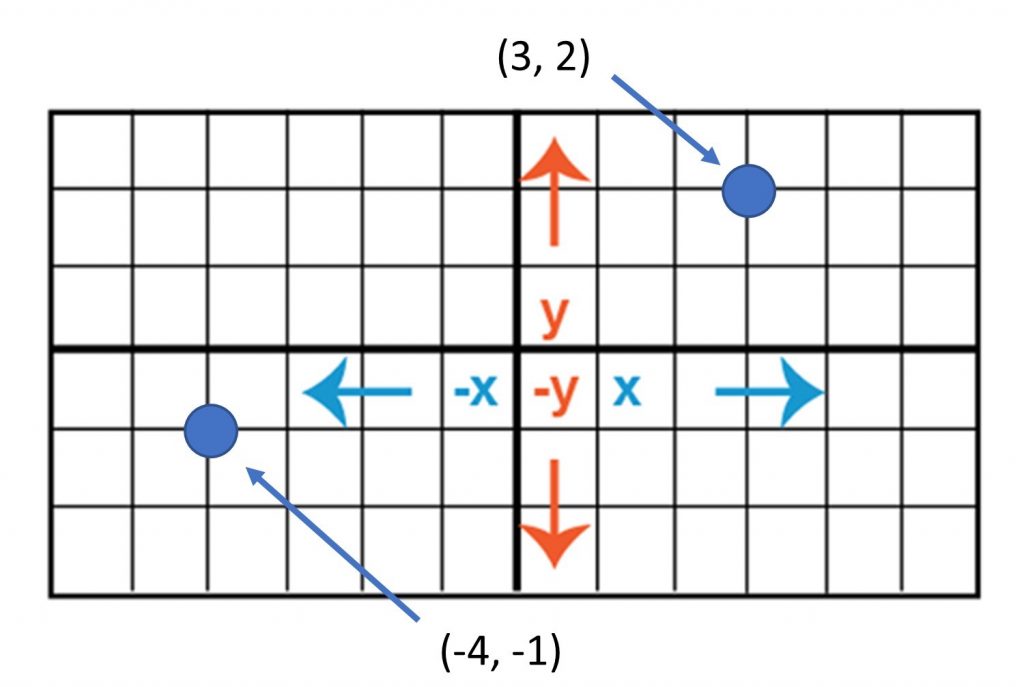
Coordinate arrangement. Locations on the Earth's surface are measured in terms of coordinates, a ready of ii or more numbers that specifies a location in relation to some reference organisation. ^[5]

The geographic coordinate organisation is designed specifically to ascertain positions on the Earth's roughly-spherical surface. Instead of the two linear measurement scales 10 and y, every bit with a Cartesian filigree, the geographic coordinate system uses an east-westward scale, called longitude that ranges from +180° to -180°. Because the World is round, +180° (or 180° East) and -180° (or 180° W) are the same filigree line, termed the International Date Line. Reverse the International Date Line is the prime peak, the line of longitude defined equally 0°. The north-southward scale, called latitude, ranges from +90° (or xc° N) at the North pole to -ninety° (or 90° S) at the South pole. In uncomplicated terms, longitude specifies positions east and west and breadth specifies positions n and south. At higher latitudes, the length of parallels decreases to goose egg at 90° Due north and South. Lines of longitude are not parallel, merely converge toward the poles. Thus, while a degree of longitude at the equator is equal to a distance of about 111 kilometers, that altitude decreases to aught at the poles.

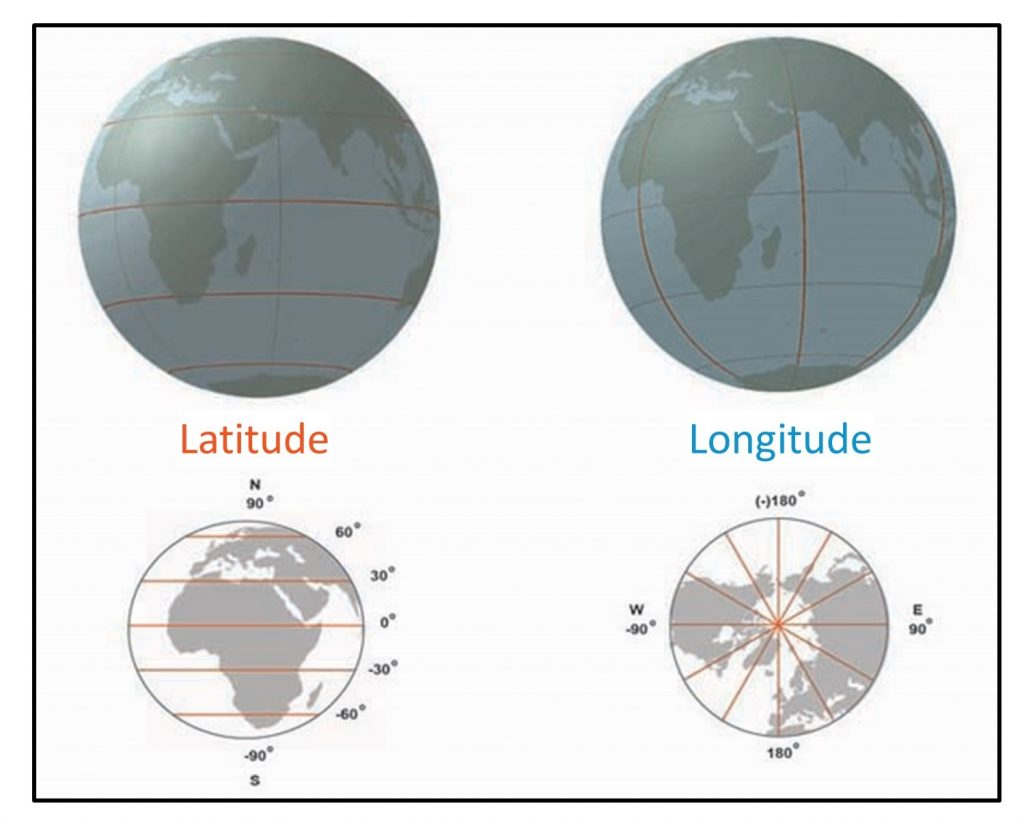
Longitude and latitude. The graticule is based on an eastward-west scale called longitude and a north-southward scale chosen latitude. ^[6]

The graticule specifies positions on the world with latitude and longitude coordinates. The graticule refers to longitude and latitude on a three-dimensional globe. When we use longitude and breadth on a ii-dimensional map, nosotros refer to these as geographic coordinates. Maps can have an enormous array of different coordinate systems depending on who developed and used them.

Projection is the term for turning a 3-dimensional globe into a 2-dimensional map. Every bit noted to a higher place, the graticule on a globe is helpful, but how do we go from three-dimensional graticule to two-dimensional geographic coordinates, as per the figure below? We will discuss the process of how objects on a 3-dimensional surface (the globe) come to be represented on a apartment piece of paper or computer screen. Our emphasis will be on the properties that different projections distort or maintain – area, shape, and distance.

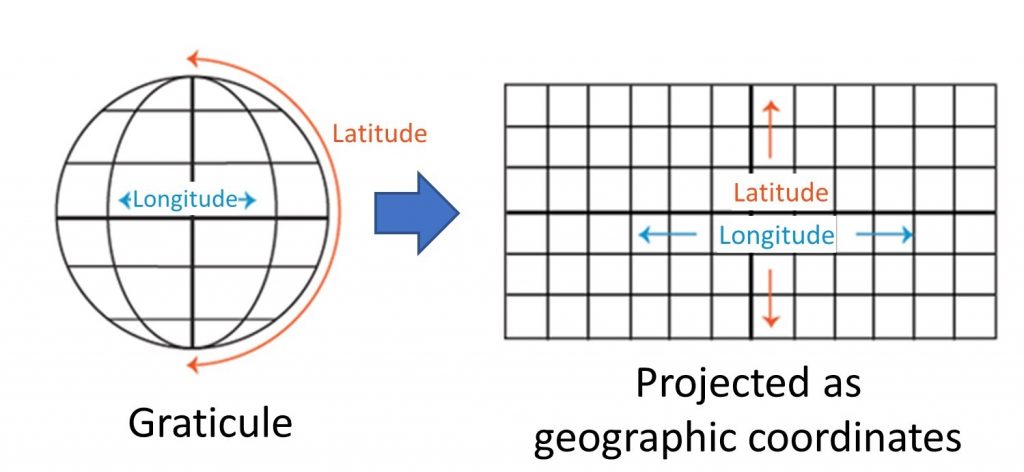
Graticule projected. The longitude and latitude of the graticule become 2-dimensional geographic coordinates through projection. ^[7]

Project is the process of making a two-dimensional map from a three-dimensional globe. We tin can recall of the earth as a sphere. In reality, it is more of an ellipsoid with a few bulges, but it is fine to think of it every bit a sphere. To become a sense of how difficult this process can be, imagine peeling the skin from an orange and trying to lay the skin flat.

Flattened orange pare. You tin can imagine the difficulty of moving from a 3d to 2d surface past considering how difficult it is to pare the rind from an orange and endeavor to lay the skin flat. ^[eight]

Equally you peel and flatten the pare, you will encounter several problems:

• Shearing – stretching the skin in one or more than directions
• Tearing – causing the skin to separate
• Compressing – forcing the peel to bunch up and condense

Cartographers face the same iii problems when they try to transform the three-dimensional earth into a two-dimensional map. If you had a globe made of paper, yous could carefully attempt to 'peel' it into a flat piece of newspaper, but you would accept a big mess on your hands. Instead, cartographers use projections to create useable two-dimensional maps.

Shearing, vehement, compression. Cartographers face these three bug of shearing, violent, and compression on a globe when they attempt to transform the three-dimensional globe into a two-dimensional map. ^[9]

The term "map projection" refers to both the process and production of transforming spatial coordinates on a 3-dimensional sphere to a two-dimensional aeroplane. In terms of bodily mechanics, most projections use mathematical functions that take every bit inputs locations on the sphere and translate them into locations on a two-dimensional surface.

It is helpful to think near projections in concrete terms. If you had a clear globe the size of a beach ball and placed a light inside this globe, it would cast shadows onto a surrounding surface. If this surface were a piece of paper that you wrapped effectually the globe, y'all could carefully trace these shadows onto the newspaper, then flatten out this piece of paper and accept your project!

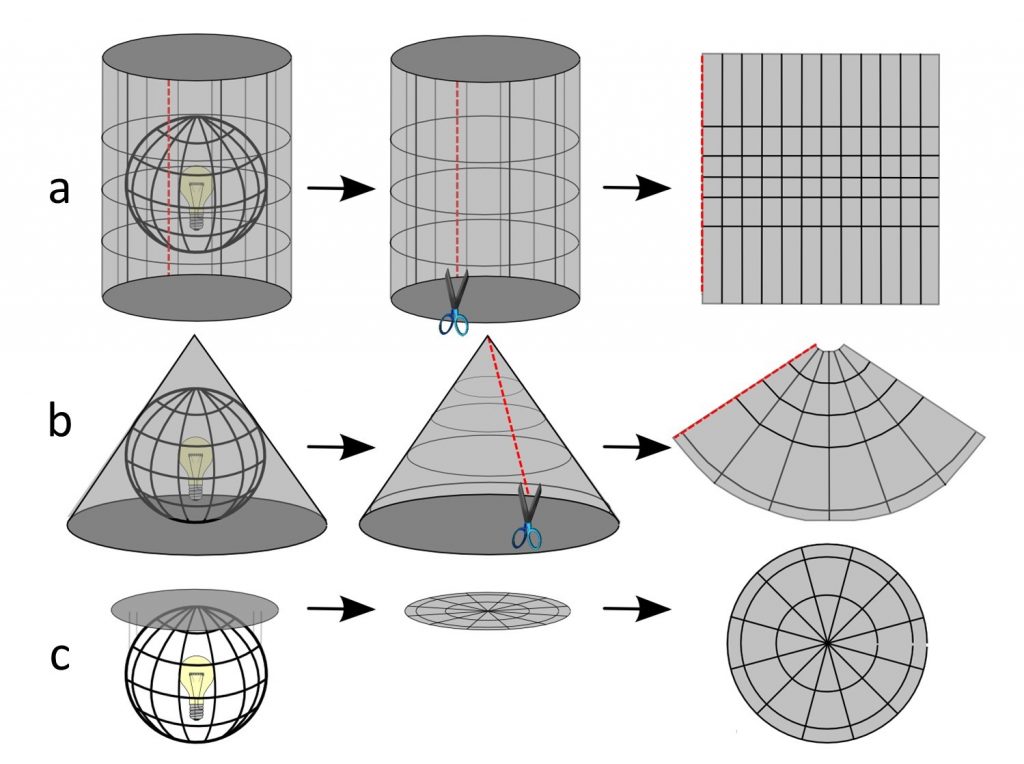
Thinking of projections in concrete terms. Yous can anticipate projection as working with a clear earth, a light bulb, and tracing paper. If you had a articulate globe the size of a beach ball and placed a calorie-free within this globe, it would bandage shadows onto a surrounding surface. This surface can be a (a) cylinder, (b) cone, or (c) plane. ^[10]

Most projections transform part of the globe to ane of iii "developable" surfaces, so called considering they are flat or can be made flat: airplane, cone, and cylinder. The resultant projections are chosen planar, conical, and cylindrical. Nosotros utilise developable surfaces considering they eliminate trigger-happy, although they will produce shearing and pinch. Of these three problems, violent is seen as the worst considering you would exist making maps with all sorts of holes in them! As nosotros see below, however, there are times when you can create maps with violent and they are quite useful.

The place where the developable surface touches the globe is known every bit the tangent point or tangent line. Maps volition nearly accurately represent objects on the globe at these tangent points or lines, with baloney increasing as you move farther abroad due to shearing and compression. It is for this reason that cylinders are frequently used for areas near the equator, cones used to map the mid-latitudes, and planes used for polar regions.

Tangency. Cherry-red lines or dots mark the tangent line or point respectively. The apartment surface touches the globe and it is the betoken on the projected map which has the least distortion. the place where the developable surface touches the globe is known as the tangent bespeak or tangent line. These surfaces can exist a (a) cylinder, (b) cone, or (c) plane. ^[xi]

For beginning mapmakers, understanding the exact mechanics of projections doesn't matter as much equally knowing which map backdrop are maintained or lost with the choice of projection – the topic of the next section.

Projections must distort features on the surface of the world during the process of making them flat because project involves shearing, tearing, and compression. Since no project tin preserve all properties, information technology is up to the map maker to know which properties are most important for their purpose and to cull an appropriate project. The properties we will focus on are shape, surface area, and distance.

Note that distortion is non necessarily tied to the type of developable surface just rather to the way the transformation is washed with that surface. It is possible to preserve any one of the iii properties using any of the developable surfaces. One way of looking at the problem is with distortion ellipses. These assistance united states to visualize what type of distortion a map projection has caused, how much distortion has occurred, and where it has occurred. The ellipses show how imaginary circles on the globe are deformed as a outcome of a detail project. If no baloney had occurred in projecting a map, all of the ellipses would be the same size and circular in shape.

3.4.ane Conformal

Conformal projections preserve shape and angle, just strongly distort expanse in the process. For instance, with the Mercator projection, the shapes of coastlines are authentic on all parts of the map, but countries most the poles appear much larger relative to countries nigh the equator than they really are. For example, Greenland is only vii-percent the country area of Africa, but it appears to be just as big!

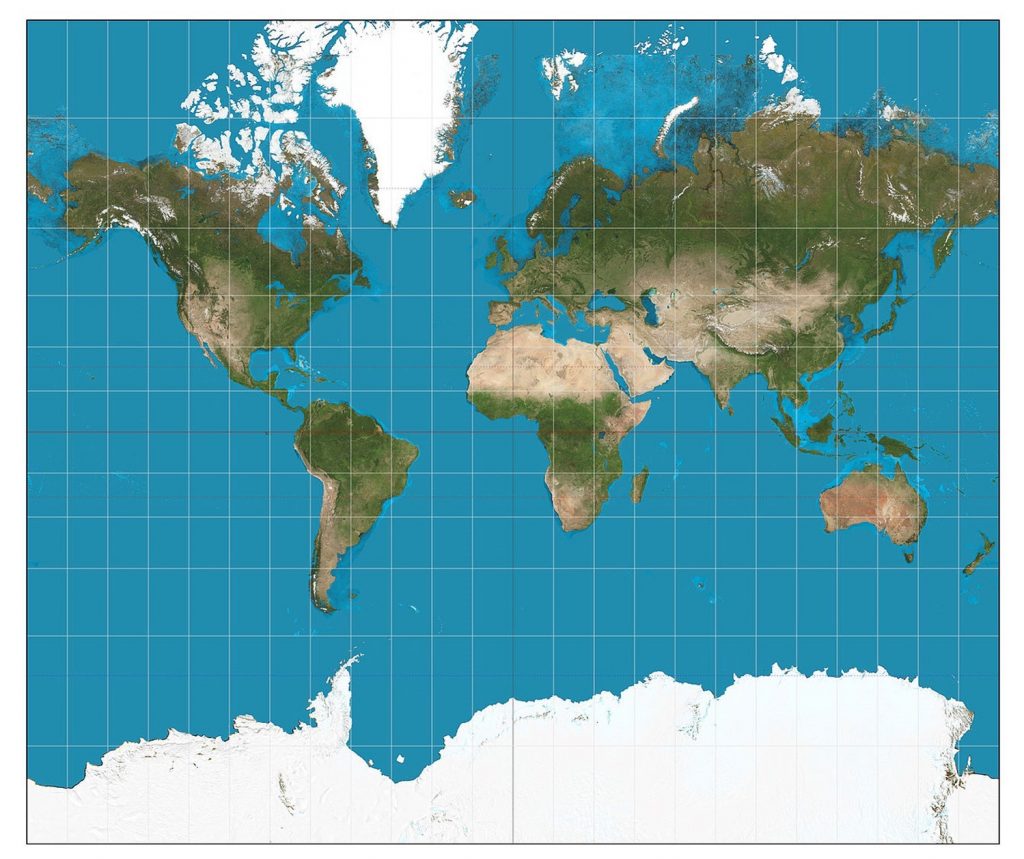
Mercator projection. The Mercator project is conformal considering it preserves shape and bending but strongly distorts area. ^[12]

Conformal projections should exist used if the main purpose of the map involves measuring angles or representing the shapes of features. They are very useful for navigation, topography (peak), and weather maps.

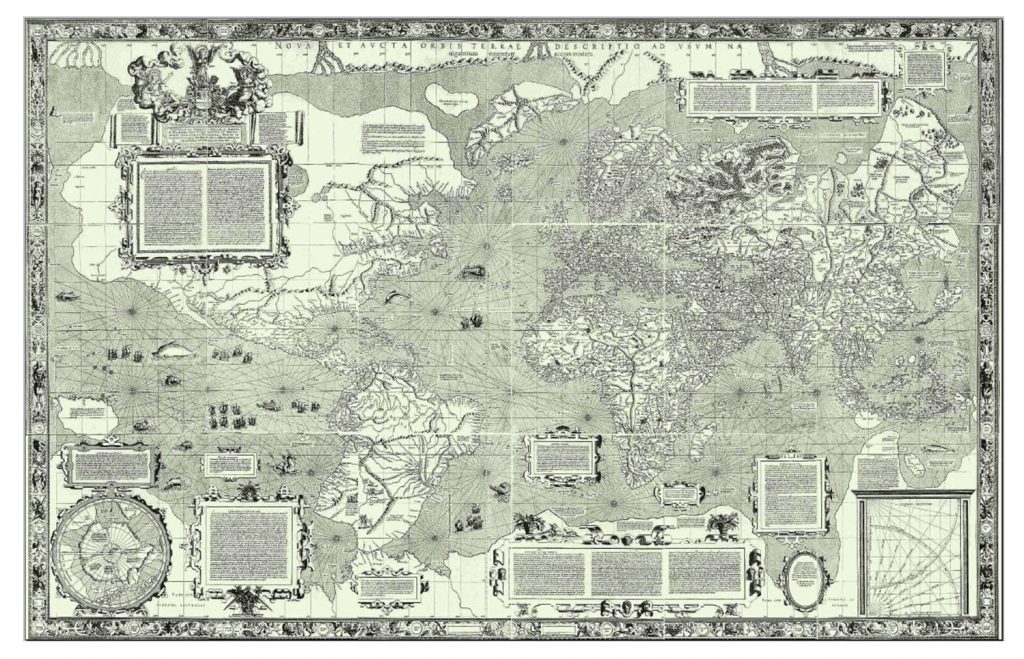
Mercator projection. Ane of the first maps of the world developed was by Mercator (Carta do Mundo de Mercator, 1569). ^[13]

A conformal projection will have distortion ellipses that vary substantially in size, but are all the same circular shape. The consequent shapes indicate that conformal projections (like this Mercator projection of the world) preserve shapes and angles. This useful property accounts for the fact that conformal projections are almost e'er used as the ground for large scale surveying and mapping.

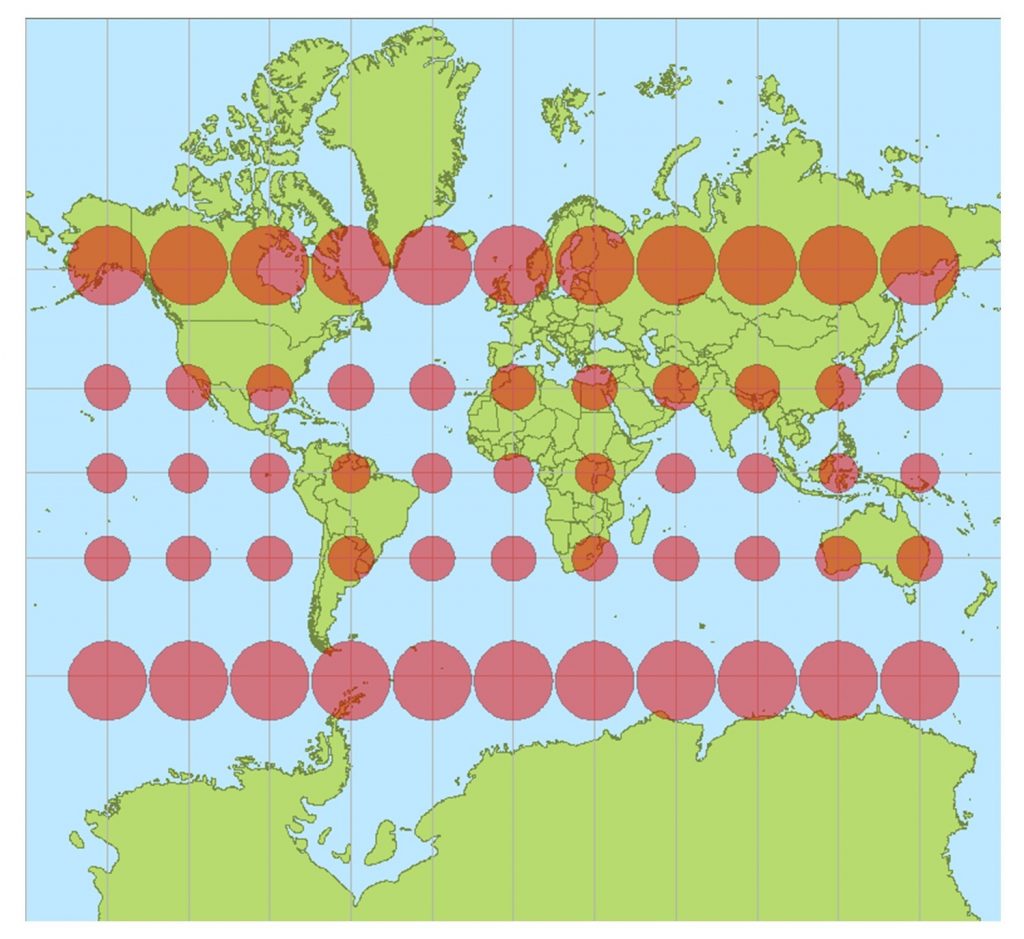
Mercator baloney. The Mercator projection is conformal because it preserves shape and angle just strongly distorts area. ^[14]

iii.4.2 Equal Surface area

On equal-area projections, the size of whatsoever area on the map is in true proportion to its size on the earth. In other words, countries' shapes may appear to be squished or stretched compared to what they look similar on a world, only their land area volition exist accurate relative to other land masses. For example, in the Gall-Peters projection, the shape of Greenland is significantly contradistinct, only the size of its area is right in comparison to Africa. This blazon of projection is of import for quantitative thematic information, especially in mapping density (an attribute over an area). For instance, it would be useful in comparing the density of Syrian refugees in the Heart E or the corporeality of cropland in production.

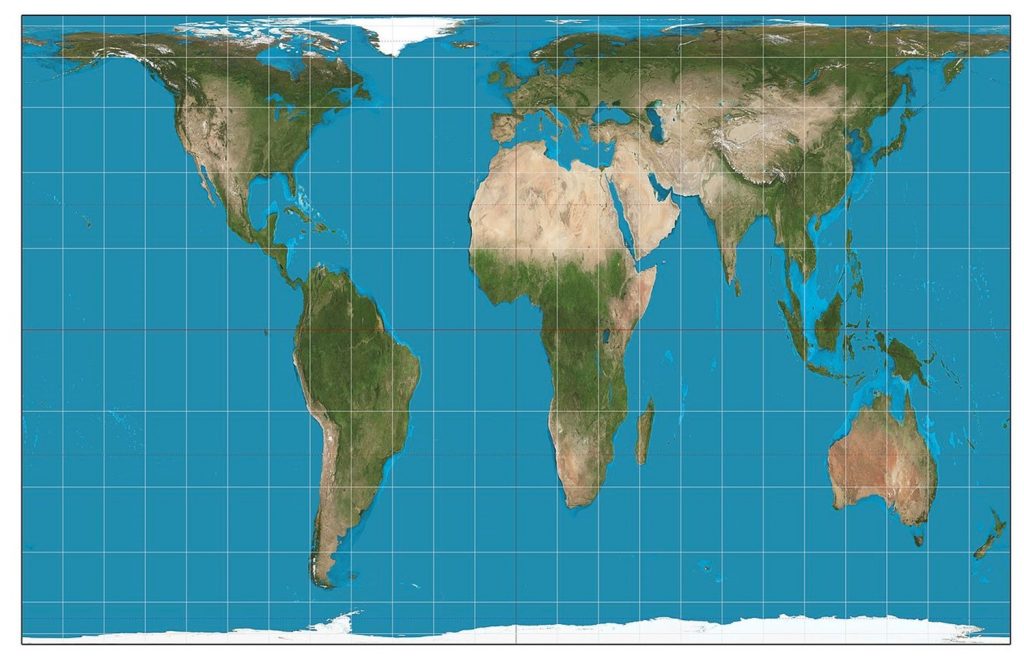
Gall-Peters project. The Gall Peters projection is equal area. Note how the shape of Greenland is significantly contradistinct, but the size of its surface area is correct in comparing to other regions such as Africa. ^[fifteen]

Every bit we can meet with an equal-area projection, however, the ellipses maintain the correct proportions in the sizes of areas on the globe but that their shapes are distorted. Equal-area projections are preferred for small-scale thematic mapping, specially when map users are expected to compare sizes of area features like countries and continents.

Gall-Peters distortion. The Gall Peters project is equal area. Note how the shape of Greenland is significantly altered, but the size of its surface area is correct in comparison to other regions such equally Africa. ^[sixteen]

iii.four.3 Equidistant

Equidistant projections, as the name suggests, preserve altitude. This is a bit misleading because no projection can maintain relative distance between all places on the map. Equidistant maps are able, however, to preserve distances along a few clearly specified lines. For example, on the Azimuthal Equidistant projection, all points are the proportionally correct altitude and direction from the center point. This type of projection would exist useful visualizing aeroplane flight paths from one city to several other cities or in mapping an earthquake epicenter. Azimuthal projections preserve altitude at the cost of distorting shape and area to some extent.  The flag of the Un contains an example of a polar azimuthal equidistant projection.

Azimuthal Equidistant projection. In this equidistant projection, all points are the proportionally correct altitude and direction from the eye point. This projection is used on the map of the United nations. ^[17]

3.4.4 Compromise, Interrupted, and Artistic Projections

Some projections, including the Robinson project, strike a residue between the different map backdrop. In other words, instead of preserving shape, area, or distance, they try to avert farthermost distortion of whatever of these properties. This type of project would be useful for a general purpose world map.

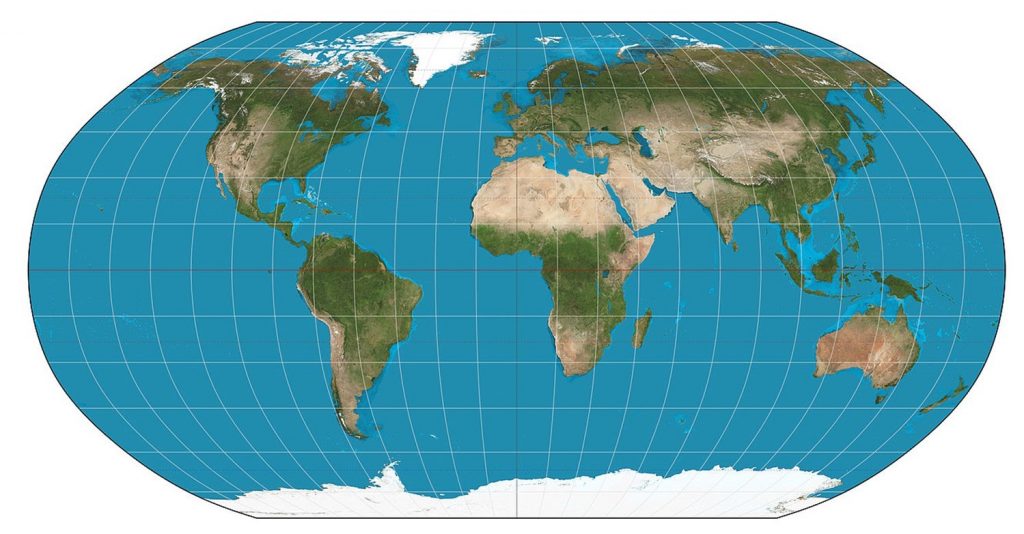
Robinson Projection. Some projections, including the Robinson project, strike a balance between the different map properties. In other words, they practice not preserve shape, expanse, or distance, merely instead effort to avoid extreme distortion. ^[18]

Compromise projections preserve no one property merely instead seek a compromise that minimizes distortion of all kinds, as with the Robinson projection, which is often used for small-scale thematic maps of the earth.

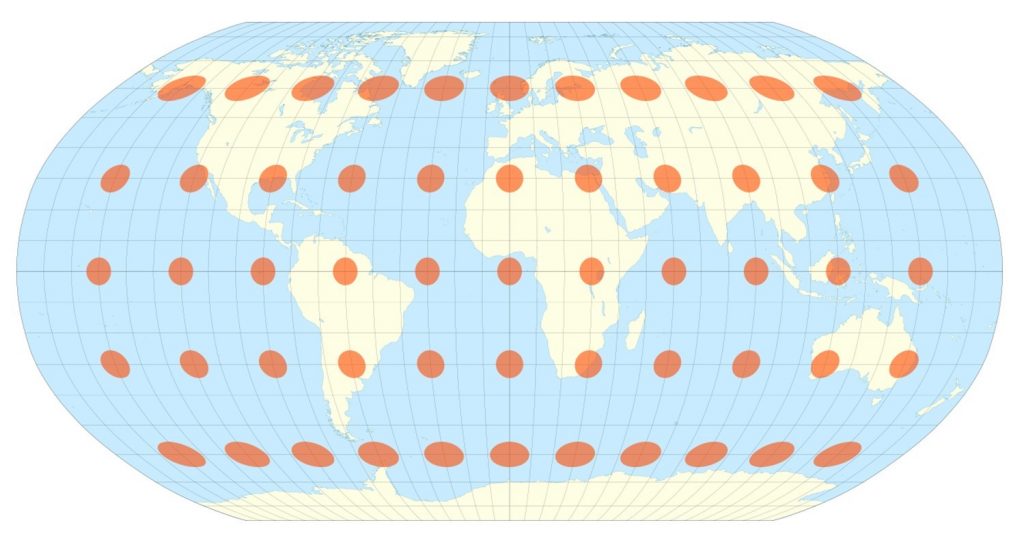
Compromise distortion. Note that some maps may not preserve either shape or expanse just do a pretty good job at both. ^[19]

Other projections deal with the challenge of making the 3D globe flat by tearing the earth in strategic places. Interrupted projections such as the interrupted Goode Homolosine projection represent the earth in lobes, reducing the corporeality of shape and area distortion well-nigh the poles. The projection was adult in 1923 by John Paul Goode to provide an alternative to the Mercator projection for portraying global areal relationships.

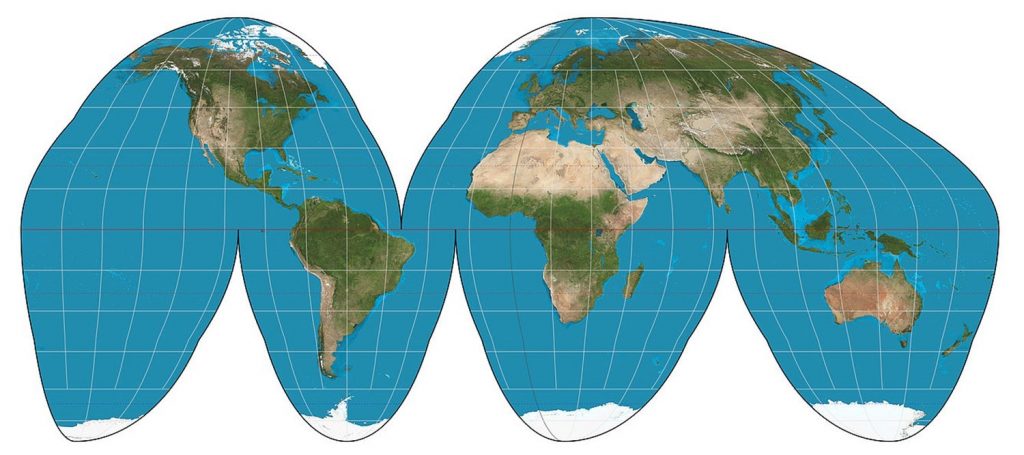
Goode homolosine projection of the globe. This equal-surface area project is interrupted in the sense that it uses lobes or sections. ^[20]

The Interrupted Goode Homolosine preserves area (and so it is equal-area or equivalent) merely does non preserve shape (it is not conformal).

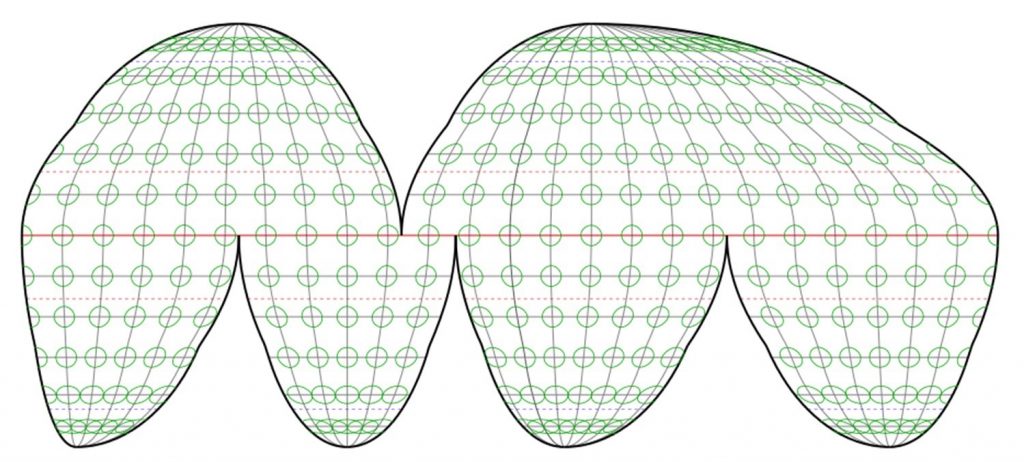
Interrupted baloney. This equal area projection preserves area but distorts shape, but not as much equally it would if it were not interrupted. ^[21]

There are likewise many projections that are aesthetically pleasing, but not intended for navigation between places or to visualize information. Examples of these artistic projections include the middle-shaped Stabius-Werner projection.

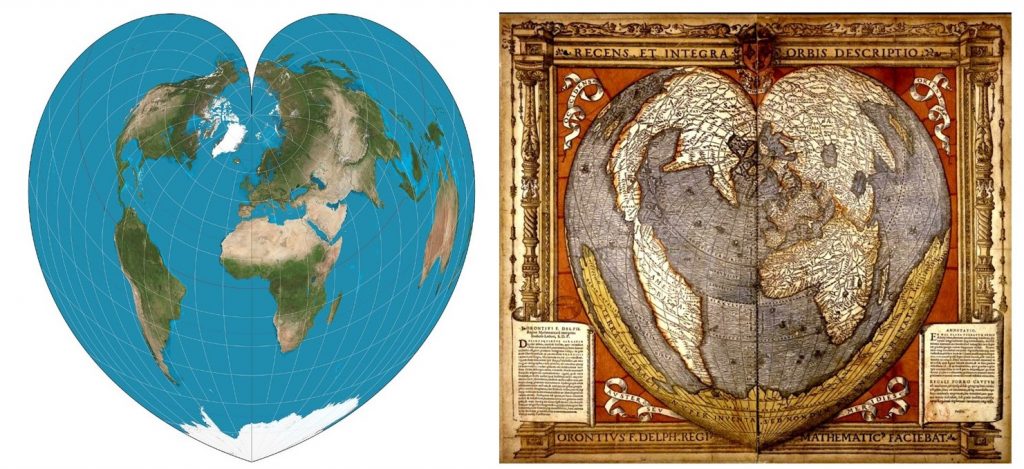
Creative projections. Many projections are interesting and cute, like this Stabius-Werner Projection, only are not intended for navigation between places or to visualize information. ^[22]

In this chapter, we have explored the concepts of scale, resolution, and projection. At that place are hundreds of projections, each which distorts the world in a slightly dissimilar manner. Continue in mind that all maps have a calibration and at that place are a few important ways to indicate this scale. All maps likewise utilize a projection that tin be formed from a developable surface and tin preserve one or two properties at most.

Resources

• GIS Commons
• Spatially Integrated Social Science

References

Parts of section 3.1 are adapted from Campbell and Shin (2011). Essentials of Geographic Information Systems.

Parts of section 3.3 are adjusted from DiBiase (1998). The Nature of Geographic Information: An Open Geospatial Textbook.

---

Source: https://open.lib.umn.edu/mapping/chapter/3-scale-and-projections/

Posted by: vanalstynerefustoo.blogspot.com

Share this post